Lambert-W step-potential

Computational physics

Numerical analysis · Simulation Data analysis · Visualization
Potentials Morse/Long-range potential · Lennard-Jones potential · Yukawa potential · Morse potential
Fluid dynamics Finite difference · Finite volume Finite element · Boundary element Lattice Boltzmann · Riemann solver Dissipative particle dynamics Smoothed particle hydrodynamics Turbulence models
Monte Carlo methods Integration · Gibbs sampling · Metropolis algorithm
Particle N-body · Particle-in-cell Molecular dynamics
Scientists Godunov · Ulam · von Neumann · Galerkin · Lorenz

The Lambert-W step-potential^[1] affords the fifth – next to those of the harmonic oscillator plus centrifugal, the Coulomb plus inverse square, the Morse, and the inverse square root^[2] potentials – exact solution to the stationary one-dimensional Schrödinger equation in terms of the confluent hypergeometric functions.^[3] The potential is given as

V(x) = \frac{V_0}{1+W (e^{-x/\sigma})}

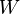where $W$ is the Lambert function also known as the product logarithm. This is an implicitly elementary function that resolves the equation $We^W=z$ .

The Lambert 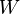$W$ -potential is an asymmetric step of height 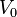$V_0$ whose steepness and asymmetry are controlled by parameter 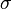$\sigma$ . If the space origin and the energy origin are also included, it presents a four-parametric specification of a more general five-parametric potential which is also solvable in terms of the confluent hypergeometric functions. This generalized potential, however, is a conditionally integrable one (that is, it involves a fixed parameter).

Solution

The general solution of the one-dimensional Schrödinger equation for a particle of mass 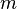$m$ and energy $E$ :

\frac{d^2\psi}{dx^2}+\frac{2m}{\hbar^2}(E-V(x))\psi=0

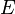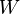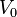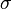for the Lambert $W$ -barrier for arbitrary $V_0$ and $\sigma$ is written as

\psi(x)=z^{i\delta/2}e^{-isz/2}\left(\frac{du(z)}{dz}-i\frac{\delta+s}{2}u(z)\right), z=W(e^{-x/\sigma})

where $u(z)$ is the general solution of the scaled confluent hypergeometric equation

u''(z)+\left(\frac{i\delta}{z}-is\right)u'(z)+\frac{as}{z}u(z)=0

and the involved parameters are given as

a=\frac{\delta(\delta+s)}{2s}+\frac{\sigma\sqrt{m}V_0}{\sqrt{2E}\hbar}, \delta=2\sigma\sqrt{\frac{2m(E-V_0)}{\hbar^2}}, s=2\sigma\sqrt{\frac{2mE}{\hbar^2}}

A peculiarity of the solution is that each of the two fundamental solutions composing the general solution involves a combination of two confluent hypergeometric functions.

If the quantum transmission above the Lambert 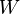$W$ -potential is discussed, it is convenient to choose the general solution of the scaled confluent hypergeometric equation as

u=c_1(isz)^{1-i\delta}{}_1F_1(1+i(a-\delta);2-i\delta;isz)+c_2 U(ia;i\delta;isz)

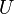where $c_{1,2}$ are arbitrary constants and ${}_1F_1$ and $U$ are the Kummer and Tricomi confluent hypergeometric functions, respectively. The two confluent hypergeometric functions are here chosen such that each of them stands for a separate wave moving in a certain direction. For a wave incident from the left, the reflection coefficient written in terms of the standard notations for the wave numbers

k_1=\sqrt{\frac{2mE}{\hbar^2}},k_2=\sqrt{\frac{2m(E-V_0)}{\hbar^2}}

reads

R=e^{-2\pi\sigma k_2}\frac{\sinh{\left(\frac{\pi \sigma}{2 k_1}(k_1-k_2)^2\right)}}{\sinh{\left(\frac{\pi \sigma}{2 k_1}(k_1+k_2)^2\right)}}

References

This article is issued from Wikipedia - version of the 10/8/2015. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.

Lambert-W step-potential

Solution

See also

References